Beam Theory in Sandwich Panel Design

Beam Theory for Sandwich Panels

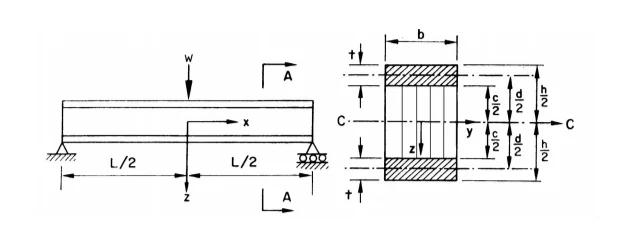
Outlining the elastic analysis of the sandwich beams in considering the three-point bending are discussed in this section in which proper evaluation of the stresses in core or skin through various mechanism are developed well.

Here,

L is the span of sandwich beams

B is width of sandwich beams

which are loaded with central load W per unit width. This is illustrated in Fig 2.1.

T is the thickness of the skin and it is supported by a thick layer which is honeycomb core of the thickness C.

D=Efxbt3/6+Efxbtd2/2+Ecxbc3/12 (2.1)

It is necessary to develop proper assumptions which are,

Skin is firmly bonded to core

Beam bends in a cylindrical manner with no curvature in the yz plane

The cross section remain plane and it is perpendicular to the axis of the beam.

D is the flexural rigidity of the sandwich beam which is developed as,

Here,

d is the distance between the upper and bottom skin of the mid-planes.

Ef x and Ecx are Young’s moduli of the skin and core respectively.

X is the direction along the axis.

f is facial material.

c is the honeycomb core.

s is solid material through which honeycomb is made of.

For simplifying the equation, it needs to be assumed that, bending of skin about centroid of the beam is dominant term and the third and first term amounts about less than 1% of this, when,

‘I’ is the second moment of the areas of cross section under the sandwich beam.

M is the maximum bending moment and corresponding stress in the skin is developed as,

The above model lacks to describe the effects of shear deflection in the core which further become appropriate for the low-density core. Proper inclusion of the model is effective for observing differences in the sandwich beams, orientations and its strengths as well as strength of the honeycomb ribbon [Refer to section 2.2.3]. the suggestion of Allen [31] for maximum axial stresses is evaluated further,

Gcxz is the out of plane shear modulus of the core.

‘I’ is the second moment of area of the sandwich.

Equation 2.6 refers that, depends on the relative stiffness of the skin and the core.

Finally (2.5) results,

L is the span length or the core stiffness.

Gcxz approach infinity (2.7) tends to the simple beam model (2.4).

In this case presented in section 2.3.1, maximum deviations from the simple beam model occurs for the fact that the finite thickness of the skins and the effect of finite shear stiffness in the core amount is to be a maximum of 26%.

Skin Failure

Section 2.2 refers an expression for the maximum stress in the skins.

The skin failure modes of face yielding intra-cell dimpling or face wrinkling are illustrated in Fig 2.2.

Face Yielding

Equation [2.5] is effective to describe in-plane strength of the face material along with the axis and failure occurs in the top skin when axial stress is there.

It is assumed here that; the skin behaves in a brittle manner. in the symmetrical beam, the stress is the same in tension and compression faces and for composite face materials, the compression face becomes critical one.

Intra-cell Dimpling

Buckling the face is the reason when the honeycomb core may fail and the wall are not supported well, illustrated in fig 2.2.b. in this context simple elastic plate buckling theory is utilised to derive expression for the stress in plane at which intra-cell buckling occurs as described below,

where, is the cell size (i.e. the diameter of the inscribed circle) of the honeycomb.

and are the elastic modulus and Poisson’s ratio for the skin which is helpful for loading in the direction of the axis.

Kuenzi [64] and Norris [65] Equations (2.9) and (2.10), can be used to derive the value of the cell size above, where there is transition from face yielding to intra-cell buckling as,

Face Wrinkling

Face wrinkling is a buckling mode of the skin with a wavelength greater than the cell width of the honeycomb. In practice, with 3-point bending inward wrinkling of the top skin happens under the vicinity of the central load. By modelling the skin as a plate on an elastic foundation, Allen [31] provides critical compressive stress which further results in wrinkling of the top skin as,

Where is the out-of-plane Possions ratio

the out-of-plane Young’s Modulus of the honeycomb core (Refer to section 2.2.3).

Core Failure

Honeycomb sandwich structures loaded, which is in bending, can fail due to core failure. Pertinent failure modes are shear failure or indentation by local crushing in the vicinity of the loads which is illustrated in Fig. 2.3.

Core Shear

Assuming simple beam behaviour the shear stress differs through the face and core in a parabolic way, under 3-point bending. If the faces are much stiffer and thinner as compared to the core, the shear stress can be considered as linear through the face and constant in the core.

Assuming that, brittle behaviour failure happens, when the applied shear stress equals to the shear strength of the honeycomb core in this direction.

Low density Nomex cores are particular susceptible in this failure mode due to the anisotropy of the honeycomb structure (refer to section 2.2.3) and the shear strength of the core relies on the loading direction.

Local Indentation

Due to local indentation, there happens failure of sandwich panel in 3 point bending and this failure is or the reason that there is crushing under the indenter. The bending stiffness both in skin and core determine the degree of load which is spread out at the point of application. In this regard, the skin wrinkle differs from indentation. In indentation the top skin deflects after failure with a wavelength of the same scale as the indenter-top skin contact length, where in skin wrinkling the deflection of the top skin after failure exhibits wavelengths, that are larger as compared to the contact length between the indenter and the top skin. Indentation failure has not been developed for honeycomb sandwich panels. In order to include this important failure mechanism, it is effective to utilise simple empirical approach in the sandwich panel construction [53]. Here, it needs to be assumed that, the length of contact between the central roller and the top skin is significant. It is further assumed that, the load is transferred to the core over this contact length, and in this case the out-of-plane compressive stress in the core is described by,

This length is derived from the assumption that, the skins are “transparent” to equate the contact length with the length of initial damage in the top skin-core interface. the above approaches have three aspects such as,

(i) The contact area must be estimated in some way in the experiments described in section 2.4

(ii) Load transfer from the roller to the core is over-simplified and it depends on the core stiffness and relative skin

(iii) Failure in the core is not be governed solely by the compressive stress in the core but it is also influenced by the local shear stress.

Honeycomb Mechanic

In order to describe the failure mechanisms, described in section 2.2, stiffness and strength properties for the honeycomb core are necessary to be analysed. In this section, it is important to use the results of references [25, 27] for expressing the properties of the honeycomb as a function of the properties of the solid material, from which the honeycomb is made of. Although the model and concept are applicable to any honeycomb in practice, by focusing on the Nomex honeycomb core, used in the beam failure experiments of section 2.4. The following notations “1”, “2”, “3” are established for honeycomb’s main axes refer to those illustrated in Fig. 1.3. The honeycomb Poisson’s ratio vcxz is required for analysing the failure (section 2.2.1) or for in plane Poisson strains, due to out of plane loading in the 3 direction. To a first approximation, its value can be considered as that of the solid material (eq. (4.64) in ref. [25] i.e. v13=v23=vs. The Young’s modulus of the honeycomb in the out of plane 3 direction is specified by the rule of mixture expression.

cc is the relevant collapse strength that can be simply estimated by using the rule of mixtures expression.

ccsc=c/s, where sc is the compressive strength of the solid from which the core is made. For Nomex honeycombs, failure is due to crushing mechanism and it can be initiated by elastic buckling and plastic buckling process. Wierzbicki [66] develops an alternative expression for the failure stress, which is based on a plastic collapse model. Under the honeycomb, regular hexagonal cells approach forecasts the collapse strength.

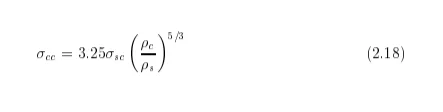
Zhang and Ashby [27] show that, the out-of-plane shear strength and stiffness of honeycombs are autonomous of height and cell size. Honeycomb cores display slight anisotropy in the out-of-plane shear strength and stiffness. It is due to the set of doubled walls which can be possible to conduct by using simple mechanics models. The simple mechanism model in this regard is based on an array of regular hexagons by considering the double wall effect. In this regard, the shear strengths 31 and 32 are derived as

And for the shear modulus G31and G32 as

The equation (2.6) refers to the core shear modulus Gcxz , which is effective to calculate the skin stress, that should be taken as either G31or G32. This further depends on the orientation of the ribbon, direction in the honeycomb. Similarly, the core shear strength cs depends on the honeycomb orientation.